What is the Regents Mathematics Reference Sheet?
For each Regents End of Course exam in mathematics, students have access to the official “High School Math Reference Sheet” for the duration of the assessment. This Regents Mathematics reference sheet provides students with the formulas and equations they need to know for the Algebra 1, Algebra 2, and Geometry Regents exams.
However, this isn’t an all-encompassing cheat sheet. Having access to the formulas is not enough to ace the test. Students need to know how to use the mathematical formulas in the context of their Regents questions.
That’s why we’ve created a guide for what students need to remember and practice to best use the Regents formula sheet. Check it out!
Regents Mathematics Formulas
We’ve compiled the formulas from the Regents mathematics equation sheet. Click on the formulas in the table below to see our expert breakdown of each formula in action.
We’ve outlined the terms of each formula, Regents questions that assess student understanding of the formula, and some questions you can use to practice the formula on Albert.
| Triangle | A=\dfrac{1}{2}bh | Pythagorean Theorem | a^2+b^2=c^2 |
| Parallelogram | A=bh | Quadratic Formula | x=\dfrac{-b\pm\sqrt{b^2-4ac}}{2a} |
| Circle | A=\pi{r}^2 | Arithmetic Sequence | a_n=a_1+(n-1)d |
| Circle (2) | C=\pi{d} or C=2\pi{r} | Geometric Sequence | a_n=a_1{r}^{n-1} |
| General Prisms | V=Bh | Geometric Series | S_n=\dfrac{a_1-a_1{r^n}}{1-r}\text{ where }r\neq1 |
| Cylinder | V=\pi{r}^2h | Radians | 1\text{ radian}=\dfrac{180}{\pi}\text{ degrees} |
| Sphere | V=\dfrac{4}{3}\pi{r}^3 | Degrees | 1\text{ degree}=\dfrac{\pi}{180}\text{ radians} |
| Cone | V=\dfrac{1}{3}\pi{r}^2h | Exponential Growth/Decay | A=A_{0}e^{k(t-t_0)}+B_0 |
| Pyramid | V=\dfrac{1}{3}Bh | ||
Regents Mathematics Formula Guide
Area of a Triangle Formula
A=\dfrac{1}{2}bh
What to Know
- A represents the area of a triangle
- b represents the base of a triangle
- h represents the height of a triangle, which is the distance from the base to the opposite vertex
Most often appears in the Regents Geometry exam

When to Use
Use this formula when you know (or are able to calculate) two out of the following three attributes of a triangle: the base, the height, and the area. You can use the formula to find the third attribute.
Example question:
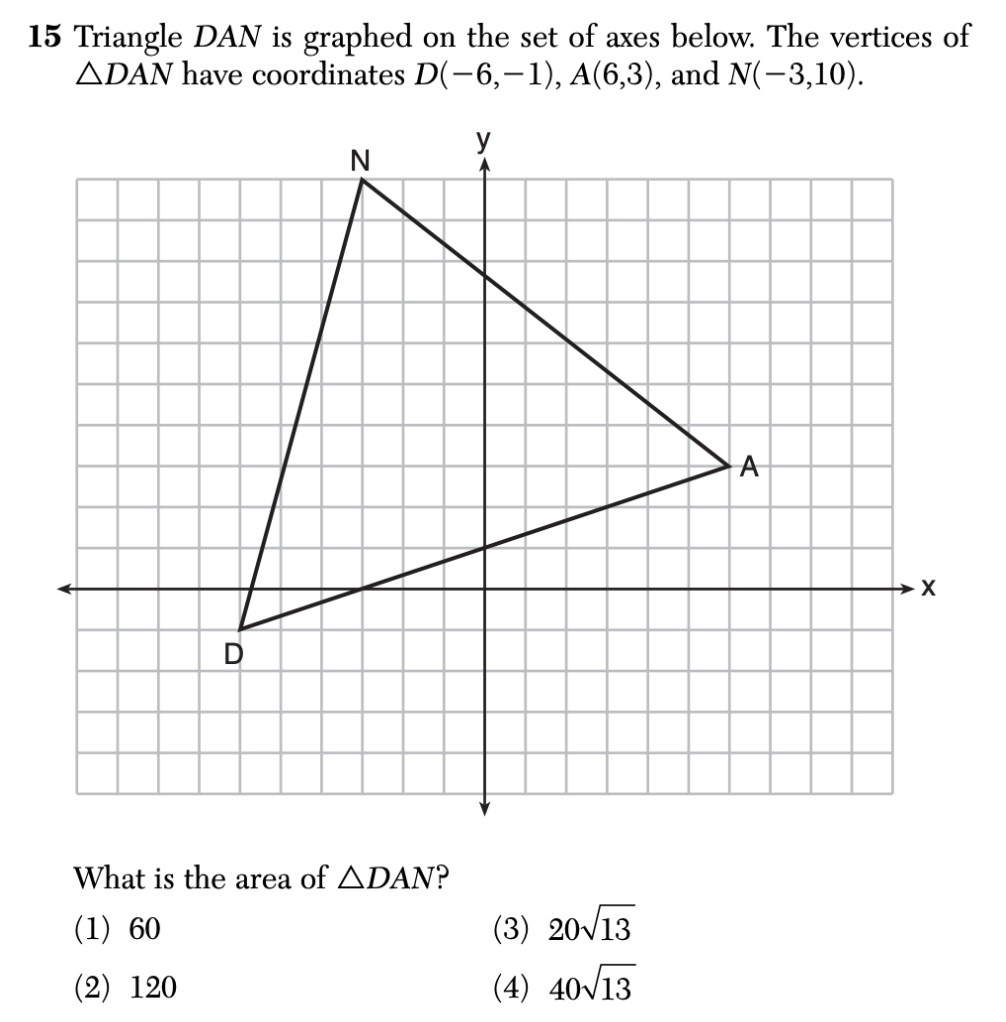
Source: June 2018 Geometry Regents, Question 15
In this problem, we would use the triangle area formula because we can calculate the base and height using the distance formula and/or Pythagorean Theorem. Then, we can substitute these values into the area formula to calculate the area using multiplication.
In this case, the base (\overline{DA}) is 4\sqrt{10}\text{ units} long and the height (from the midpoint of \overline{DA} to N) is 3\sqrt{10}. We can substitute these values into the area formula to find the area:
A=\dfrac{1}{2}bh
A=\dfrac{1}{2}\cdot4\sqrt{10}\cdot3\sqrt{10}
A=60
So, the area of this triangle is 60. Option 1 is correct.
Try it Yourself
Check out these practice problems on Albert so that you can try out the area of a triangle formula for yourself:
- Foundations of Algebra / Area of Polygons / Level 1: Area of right triangles (free)
- Foundations of Algebra / Area of Polygons / Level 2: Area of all triangles (free)
- Geometry / Area and Perimeter / Level 1: Calculating area of triangles
Return to the Top
Area of a Parallelogram Formula
A=bh
What to Know
- A parallelogram is a four sided polygon with opposite sides parallel
- Types of parallelograms include squares, rectangles, and rhombuses
- A represents the area of a parallelogram
- b represents the base of a parallelogram
- h represents the height of a parallelogram, which is the vertical distance from the base to the opposite side
- Most often appears in the Regents Geometry exam

When to Use
Use this formula when you know or are able to calculate two of the following three attributes of a parallelogram: its height, its base, and its area. You can use the formula to find the missing attribute.
Example question:

Source: January 2020 Geometry Regents, Question 18
Since a rhombus is a type of parallelogram, we can use the area formula for parallelograms to solve this problem. We aren’t explicitly given the height and base of the parallelogram, but we can use the distance formula and/or Pythagorean Theorem to find them.
In this case, the base is the length of \overline{CD}, which is equal to 5\text{ units}. The height is the distance between \overline{CD} and \overline{AB}, which is also equal to 5\text{ units}. We can find the area with the formula:
A=bh
A=5\cdot5
A=25
So, the area of rhombus ABCD is 25 and Option 3 is correct.
Try it Yourself
Check out these practice topics from Albert’s Geometry course:
- Geometry / Quadrilaterals / Level 2: Squares
- Geometry / Quadrilaterals / Level 3: Rectangles
- Geometry / Quadrilaterals / Level 4: Parallelograms
Return to the Top
Area of a Circle Formula
A=\pi{r}^2
What to Know
- A represents the area of a circle
- r represents the radius of a circle, which is equal to half its diameter or the distance from the center of a circle to any location on that circle’s border
- \pi is a mathematical constant that we can approximate as 3.14
- Most often appears in the Regents Geometry exam

When to Use
Use the formula when you know or are able to calculate either the area or radius of a given circle and when you want to find the other attribute. Recall that the length of a circle’s radius is equal to one half its diameter, so knowing a circle’s diameter is enough to find its area.
Example question:

Source: June 2017 Geometry Regents, Question 26
This is a multistep problem that ultimately asks us to find the area of a circle’s sector, but we must start by finding the area of the given circle. The prompt indicates that the radius of the circle is 4.5, so we have everything we need to find the area.
A=\pi{r}^2
A=3.14\cdot4.5^2
A=63.585
To solve this problem, we would go on to set up a proportion that compares the 40^\circ measure of the sector to the 360^\circ in a circle and the unknown area of the sector to the total area of the circle.
\dfrac{40}{360}=\dfrac{x}{63.585}
7.065=x
So, the area of the sector is 7.065.
Try it Yourself
Put your circle skills to the test with these practice problems from Albert:
- Foundations of Algebra / Shapes: Circles / Level 3: Area of a circle (free)
- Geometry / Basic Properties / Level 2: Area of a circle
- Geometry / Areas of Sectors, Semicircles, and Segments / Level 1: Sectors
Return to the Top
Circumference of a Circle Formula
C=\pi{d} or C=2\pi{r}
What to Know
- C represents the circumference of a circle, or the distance around it
- r represents the radius of a circle, which is equal to half its diameter or the distance from the center of a circle to any location on that circle’s border
- d represents the diameter of a circle, or the longest possible chord of a circle that runs through its center
- \pi is a mathematical constant that we can approximate as 3.14
- Most often appears in the Regents Geometry exam

When to Use
Use the formula when you know either the diameter or radius of a circle and are looking to find the circumference or when you know the circumference and want to know the radius or diameter.

Source: June 2018 Geometry Regents, Question 22
While this problem is ultimately asking for the length of an arc of the given circle, we first need to find the circle’s circumference. We are given the length of the radius as 6 inches, so we can use the circumference formula. Notice that since the answer choices are in terms of \pi, we will leave \pi in our calculations.
C=2\pi{r}
C=2\cdot\pi\cdot6
C=12\pi
To finish this problem, we would now set up a proportion that compares the 120^\circ measure of the sector to the 360^\circ in a circle and the unknown length of the sector to the total circumference of the circle.
\dfrac{120}{360}=\dfrac{x}{12\pi}
\dfrac{1}{3}{(12\pi)}=x
So, Option 4 is correct.
Try it Yourself
Follow these links to practice topics on Albert to try the circumference formula for yourself:
- Foundations of Algebra / Shapes: Circles / Level 2: Circumference and pi (free)
- Geometry / Basic Properties / Level 1: Radius, diameter, circumference of circles
Return to the Top
Volume of General Prisms Formula
V=Bh
What to Know
- A prism is a three-dimensional figure with two congruent and parallel end faces
- V represents the volume of a prism
- B represents the area of a prism’s base
- h represents the height of a prism
- Most often appears in the Regents Geometry exam

When to Use
Use this formula when you want to know a prisms’s volume given its height and enough information to calculate the area of the base of that prism. This depends on the shape of the base, which might be a parallelogram or a triangle. Conversely, you can use the formula when you are given a prism’s volume and need to find another one of its measurements.
Example question:

Source: January 2020 Geometry Regents, Question 14
This question provides enough information for us to calculate the volume of a rectangular prism and then asks us to take it one step further and calculate its weight. First we need to find the area of the base of the prism, which we know is a rectangle. We can thus use the formula for area of a parallelogram, where b=8 and h=3.5.
A=bh
A=8\cdot3.5
A=28
Now we can use the formula for the volume of a prism given B=28 and h=2.25.
V=Bh
V=28\cdot2.25
V=63
So, the volume of the prism is 63 cubic inches. Since the clay weighs 1.055\text{ oz/in}^3, we can calculate the weight as 63\cdot1.055 or approximately 66 ounces. Option 1 is correct.
Try it Yourself
Get some prism practice of your own with these Albert topics:
- Foundations of Algebra / Shapes: Explore Volume / Level 2: Calculate volume with multiplication (free)
- Foundations of Algebra / Shapes: Volume and Surface Area / Level 1: Volume of right rectangular prism with fractional edge lengths (free)
- Geometry / 3D Figures – Surface Area and Volume / Prisms
Return to the Top
Volume of a Cylinder Formula
V=\pi{r}^2h
What to Know
- A cylinder is a three-dimensional figure with two congruent circular or oval end faces. This volume formula applies for right circular cylinders.
- V represents the volume of a cylinder
- r represents the radius of the circular face of a cylinder, which is equal to half its diameter or the distance from the center of a circle to any location on that circle’s border
- h represents the height of a cylinder
- \pi is a mathematical constant that we can approximate as 3.14
- Most often appears in the Regents Geometry exam

When to Use
Use this formula when you know or are able to calculate two of the following attributes of a right circular cylinder and want to know the third: the radius of the base, the height, or the total volume.
Example question:

Source: August 2019 Geometry Regents, Question 31
In this question, we are given the diameter of the base (from which we can calculate the radius as 4\dfrac{1}{8}\text{ feet}) of a right cylinder. We want to find the volume of the cylinder if it is filled to a height of 3-\dfrac{1}{2}=2\dfrac{1}{2}\text{ feet}. So, we can use the volume formula.
V=\pi{r}^2h
V=3.14\cdot{4\dfrac{1}{8}}^2\cdot2\dfrac{1}{2}
V\approx133.57
So, to the nearest cubic foot, the cubic feet of water that will fill the cylinder from the prompt is 134.
Try it Yourself
Apply the cylinder volume formula yourself with these practice problems from Albert:
- Geometry / 3D Figures – Surface Area and Volume / Cylinders
Return to the Top
Volume of a Sphere Formula
V=\dfrac{4}{3}\pi{r}^3
What to Know
- A sphere is a three-dimensional figure where every point on the surface is equidistant from the center
- Half of a sphere is called a hemisphere
- V represents the volume of a sphere
- r represents the radius of a sphere, or the distance from the center of a sphere to any location on its surface
- \pi is a mathematical constant that we can approximate as 3.14
- Most often appears in the Regents Geometry exam

When to Use
Use this formula when you know or can calculate the radius of a sphere and want to know the volume, or vice versa. Don’t forget that the radius is equal to one half of the diameter.
Example question:

Source: June 2019 Geometry Regents, Question 10
In this question, we are given the diameter of a hemisphere, which is a three-dimensional shape that comprises half of a sphere. Since the diameter of this hemisphere is 12.6\text{ cm}, we know the radius is 6.3\text{ cm}. Let’s calculate the volume of this shape as if it was an entire sphere with the same dimensions.
V=\dfrac{4}{3}\pi{r}^3
V=\dfrac{4}{3}\cdot3.14\cdot{6.3}^3
V\approx1{,}047.4
Since a hemisphere is half of a sphere, the volume of the given hemisphere would be equal to half of 1{,}047.4, or approximately 523.7. Option 1 is correct.
Try it Yourself
Put your sphere skills to the test with these practice questions on Albert:
- Geometry / 3D Figures – Surface Area and Volume / Spheres
Return to the Top
Volume of a Cone Formula
V=\dfrac{1}{3}\pi{r}^2h
What to Know
- V represents the volume of a cone
- r represents the radius of a circular base of a cone
- h represents the height of a cone, the perpendicular distance from the top to the base
- \pi is a mathematical constant that we can approximate as 3.14
- Most often appears in the Regents Geometry exam

When to Use
Use this formula when you know or can calculate two of the following three attributes of a cone and want to find the third: the radius of the circular base, the total height, and the cone’s volume. Note that this formula only works for right circular cones.
Example question:

Source: January 2018 Geometry Regents, Question 7
This prompt provides the volume of a right circular cone and the diameter of its base. From the diameter of 6.6\text{ cm}, we can calculate the radius as 3.3\text{ cm}. We can substitute the volume and radius into the volume formula and use inverse operations to find the height.
V=\dfrac{1}{3}\pi{r}^2h
54.45\pi=\dfrac{1}{3}\cdot\pi\cdot{3.3}^2\cdot{h}
15=h
So, the height of this cone is 15\text{ cm}. Option 3 is correct.
Try it Yourself
Practice applying the cone volume formula with these practice problems:
- Geometry / 3D Figures – Surface Area and Volume / Cones
Return to the Top
Volume of a Pyramid Formula
V=\dfrac{1}{3}Bh
What to Know
- A pyramid is a three-dimensional figure with a polygon base and triangular faces that meet at a point
- V represents the volume of a pyramid
- B represents the area of the base of a pyramid
- h represents the height of a pyramid, the perpendicular distance from the top to the base
- Most often appears in the Regents Geometry exam

When to Use
This formula applies only to right pyramids. Use it when you know or are able to calculate two of the following three attributes: the area of the base of a pyramid, a pyramid’s height, and a pyramid’s volume. You can manipulate the formula to find the third attribute.
Example question:

Source: August 2019 Geometry Regents, Question 21
This problem presents a right pyramid whose base is a square. We can find the area of the base like we’d find the area of any square: multiply the length of one side by another. So, the area of the base is 4{,}096\text{ cm}^2.
Note that in this problem, we are given the slant height and not the perpendicular height. We will have to employ the Pythagorean Theorem to find that the height of the pyramid is 24\text{ cm}. Now we can calculate the pyramid’s volume.
V=\dfrac{1}{3}Bh
V=\dfrac{1}{3}\cdot4{,}096\cdot24
V=32{,}768.0
The volume of the given pyramid in cubic centimeters is 32{,}768.0. Option 3 is correct.
Try it Yourself
Check out the practice problems linked below for some practice with pyramids:
- Geometry / 3D Figures – Surface Area and Volume / 3D Figures and Nets
Return to the Top
Pythagorean Theorem
a^2+b^2=c^2
What to Know
- a and b represent the legs of a right triangle, or the sides adjacent to the right angle
- c represents the hypotenuse of a right triangle, or the side across from the right angle
- Most often appears in the Regents Geometry exam

When to Use
Use the Pythagorean Theorem to find the missing side length of a right triangle given the other two side lengths.
Example question:

Source: January 2016 Geometry Regents, Question 32
We’ll have to apply several mathematical concepts to solve this problem, including the Pythagorean Theorem. Since we know the ratio of the width to the height of the given rectangle is 16{:}9, we can calculate the width to be about 36.6 from the height of 20.6. Now, the width and height make up the legs of the right triangle and the diagonal is the unknown hypotenuse.
a^2+b^2=c^2
36.6^2+20.6^2=c^2
42\approx{c}
So, the length of the diagonal is about 32\text{ in}.
Try it Yourself
Try the Pythagorean Theorem out yourself with these practice questions on Albert:
- Foundations of Algebra / Shapes: Pythagorean Theorem / Level 1: Pythagorean Theorem with right triangles (free)
- Foundations of Algebra / Shapes: Pythagorean Theorem / Level 2: Pythagorean Theorem with isosceles triangles (free)
- Foundations of Algebra / Shapes: Pythagorean Theorem / Level 3: Problem solving with Pythagorean Theorem (free)
Return to the Top
Quadratic Formula
x=\dfrac{-b\pm\sqrt{b^2-4ac}}{2a}
What to Know
- x represents the roots of the equation given by ax^2+bx+c=0 which is the same as where the graph of equation crosses the x-axis
- a represents some nonzero real number in a quadratic equation given by ax^2+bx+c=0
- b represents some real number in a quadratic equation given by ax^2+bx+c=0
- c represents some real number in a quadratic equation given by ax^2+bx+c=0
- The \pm symbol (read as “plus or minus”) indicates we can either add or subtract that portion of the formula to find a valid answer
- The quadratic formula is most often using on the Algebra 1 and Algebra 2 Regents exams
When to Use
The quadratic formula is one of the most important and useful formulas in all of math. Use the formula when trying to solve a quadratic equation in the general form:
ax^2+bx+c=0
If a quadratic equation is not in the standard form listed above, it must be rearranged before applying the quadratic formula. We can then plug the values of a, b, and c into the quadratic formula to find the “roots” (or x-intercepts) of the equation.
Example question:

Source: June 2019 Algebra 1 Regents, Question 21
We’re asked to find the roots of this equation:
x^2 - 5x -4 = 0
We can tell this equation is a quadratic because the variable, x, is being raised to the 2nd power and there are no high exponents on other terms.
There are many ways to find the roots of a quadratic, but we’re going to focus on using the quadratic formula. Since the given equation is already in standard form (ax^2+bx+c=0), we can define the the variables as follows:
a = 1
b = -5
c = -4
Now it’s time for the quadratic formula to shine! Let’s substitute the values of a, b, and c like this:
x=\dfrac{-b\pm\sqrt{b^2-4ac}}{2a}
x=\dfrac{-(-5)\pm\sqrt{(-5)^2-4(1)(-4)}}{2(1)}
Notice how we used parenthesis to make each of those substitutions. We can further simplify the right side of the equation:
x=\dfrac{5\pm\sqrt{25+16}}{2}
x=\dfrac{5\pm\sqrt{41}}{2}
We could continue simplifying our answer, but we already have our roots in the required form for this question. So, the correct answer is Option 2.
Try it Yourself
Try using the quadratic formula on your own with some practice problems from Albert. Each Albert question includes a detailed explanation:
- Algebra 1 / Solve Quadratic Equations by Using Quadratic Formula / Level 1: Solving quadratics by quadratic formula (free)
- Algebra 1 / Solve Quadratic Equations by Using Quadratic Formula / Level 2: Predicting solutions with discriminant (free)
- Algebra 2 / Quadratic Formula and the Discriminant / Level 1: Rational roots
- Algebra 2 / Quadratic Formula and the Discriminant / Level 2: Irrational roots
Return to the Top
Arithmetic Sequence
a_n=a_1+(n-1)d
What to Know
- An arithmetic sequence has a consistent amount (called the “common difference”) being added between each consecutive term
- a_n represents the n th term of the sequence
- a_1 represents the first term in the sequence
- n represents the term number we are trying to find (for example, if we’re looking for the 10th term in a sequence, n = 10)
- d represents the common difference (which is the amount being added between each consecutive term in the sequence)
When to Use
We use the arithmetic sequence formula anytime we’re trying to find a specific term in an arithmetic sequence. The formula we’re working with is called the explicit formula (there is another version of the formula called the recursive formula that is not included on the regents formula sheet).
The explicit formula for arithmetic sequences is very similar to the slope-intercept form for the equation of a line.
Example question:
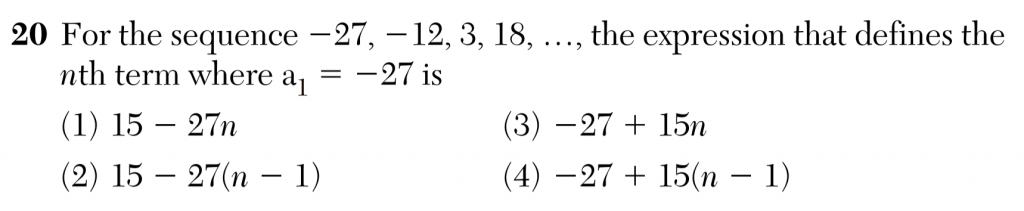
Source: August 2018 Algebra 1 Regents, Question 20
We first need to figure out what kind of sequence we are working with (arithmetic or geometric). Here is what we’re given:
-27,-12,3,18,...,
Remember: arithmetic sequences have a common difference between each term.
What can we do to get from -27 to -12? Add 15!
What can we do to get from -12 to 3? Add 15!
What can we do to get from 3 to 18? Add 15!
We can see that the sequence adds 15 between consecutive terms, so this is an arithmetic sequence!
Ok – now for the fun part 🙂 We need to represent the sequence as a formula. Let’s take a look at the arithmetic sequence formula:
a_n=a_1+(n-1)d
We’re told in the prompt that a_1 (the first term in the sequence) is -27.
Since we’re adding 15 between consecutive terms in the sequence, the common difference, d, is 15.
So, plugging in those values, we now have:
a_n=-27+(n-1)15
…which is equivalent to Option 4.
Try it Yourself
Explore arithmetic sequences on your own using the free Albert practice questions below:
- Algebra 1 / Arithmetic Sequences / Level 1: Determining common difference (free)
- Algebra 1 / Arithmetic Sequences / Level 2: Determining the nth arithmetic term (free)
- Algebra 1 / Arithmetic Sequences / Level 3: Determining an arithmetic formula (free)
Return to the Top
Geometric Sequence
a_n=a_1{r}^{n-1}
What to Know
- A geometric sequence has a consistent amount (called the “common ratio”) that is multiplied between consecutive terms
- a_n represents the nth term of the sequence
- a_1 represents the first term in the sequence
- r represents the common ratio (which is the amount being multiplied between each consecutive term in the sequence)
- n represents the term number we are trying to find (for example, if we’re looking for the 10th term in a sequence, n = 10)
- n-1 represents the term number right before the nth term (for example, if we’re looking for the 10th term in a sequence, n-1 = 9)
When to Use
We use the geometric sequence formula anytime we’re trying to find a specific term in a geometric sequence. The formula we’re working with is called the explicit formula (there is another version of the formula called the recursive formula that is not included on the regents formula sheet).
Example question:

Source: August 2019 Algebra 1 Regents, Question 24
We are told in the prompt that we’re working with a geometric sequence. We are also given the first and third terms, as defined below:
a_1 = 5
a_3 = 245
We can use the given information, along with the explicit formula for geometric sequences, to find the common ratio. We’ll start with the explicit formula for geometric sequences:
a_n=a_1{r}^{n-1}
We can substitute the known values, as such:
a_3=a_1{r}^{3-1}
245=5{r}^{2}
Now we solve for the variable, r. Divide both sides by 5.
245 \div 5 =5{r}^{2} \div 5
49 ={r}^{2}
Take the positive square root of both sides:
\sqrt{49} =\sqrt{{r}^{2}}
7 =r
The common ratio is 7. So, the correct answer is Option 1.
Try it Yourself
Explore geometric sequences on your own using the free Albert practice questions below. Each question includes a detailed explanation to help you continue improving:
- Algebra 1 / Geometric Sequences / Level 1: Determining common ratio (free)
- Algebra 1 / Geometric Sequences / Level 2: Determining the nth geometric term (free)
- Algebra 1 / Geometric Sequences / Level 3: Determining a geometric formula (free)
Return to the Top
Geometric Series
S_n=\dfrac{a_1-a_1{r^n}}{1-r}\text{ where }r\neq1
What to Know
- A geometric series is the sum of the terms in a geometric sequence
- S_n represents the sum of terms from the first term through the n th term.
- a_1 represents the first term in the sequence
- r represents the common ratio (which is the amount being multiplied between each consecutive term in the sequence)
- r \neq 1 means that the common ratio cannot be equal to 1 because then the denominator of the fraction in the geometric series formula would equal 0 (and we can’t divide by zero)
- n represents the term number we are trying to find (for example, if we’re looking for the 10th term in a sequence, n = 10)
- This formula is most often used on the Algebra 2 Regents exam
When to Use
We use the geometric series formula anytime we’re trying to find the sum of a geometric sequence. We’ll typically be given a specific number ( n ) of terms to add together and find the sum.
Example question:

Source: August 2019 Algebra 2 Regents, Question 2
We are asked to find the sum of the first 12 terms in a geometric sequence. The geometric series formula is perfect to help us with that!
Let’s first define a few of the variables we know. We’re told the first term of the sequence is 8, so:
a_1 = 8
We’re also told the fourth term is 216, so we know:
a_4 = 216
We can use the geometric sequence formula to solve for the common ratio of this sequence:
a_n=a_1{r}^{n-1}
a_4=a_1{r}^{4-1}
216=8{r}^{3}
Divide both sides by 8:
216 \div 8 =8{r}^{3} \div 8
27 ={r}^{3}
Take the cubic root of both sides:
\sqrt[3]{27} =\sqrt[3]{{r}^{3}}
3 =r
Alright – we’re making progress! We now know the common ratio (r) is 3.
Our goal is find the sum of the first 12 terms, so let’s use our handy dandy geometric series formula:
S_n=\dfrac{a_1-a_1{r^n}}{1-r}\text{ where }r\neq1
S_{12}=\dfrac{8-(8){(3)^{12}}}{1-3}
Now we evaluate the right side of the equation as follows:
S_{12}=\dfrac{8-(8){(3)^{12}}}{1-3}
S_{12}=\dfrac{8-(8)(531{,}441)}{1-3}
S_{12}=\dfrac{8-(4{,}251{,}528)}{-2}
S_{12}=\dfrac{8-(4{,}251{,}528)}{-2}
S_{12}=\dfrac{-4{,}251{,}520}{-2}
S_{12}=2{,}125{,}760
So, the final answer is Option 3.
Try it Yourself
Practice 10 Regents-aligned questions on series and sequences using the Albert topic quiz linked below. If you’re a student, ask your teacher to assign you the quiz so you can test your skills before exam day:
- Algebra 2 / Series and Sequences (Topic Quiz)
Return to the Top
Radians and Degrees
1\text{ radian}=\dfrac{180}{\pi}\text{ degrees}
1\text{ degree}=\dfrac{\pi}{180}\text{ radians}
What to Know
- Radians and degrees are both units of measurement for angles
- A full circle has 2 {\pi} radians and 360 degree
- We can use the relationships given on the reference sheet to convert between radians and degrees
- Radians and degrees are most often used on the Geometry and Algebra 2 Regents exam
When to Use
We use the relationship between radians and degrees in a variety of different problems involving angle measurements.
Example question:

Source: August 2017 Geometry Regents, Question 23
A sector is a section of a circle enclosed by two radii of a circle and their intercepted arc, like this:

We are asked to find the angle of the sector (in radians) given the following info:
d = 32
A_ \text{sector} = \dfrac{ 512 \pi}{3 }
We can solve this multiple ways. Our strategy will be to first find the area of the full circle, then find the ratio of the entire area that is represented by the sector. We can use the relationship between the sector area and the full area to find the angle.
Let’s start by finding the area of the full circle:
A_\text{circle} = \pi \left(\frac{ d}{2 }\right)^2
A_\text{circle} = \pi 16^2
A_\text{circle} = 256 \pi
Now we can take the area of the sector and divide by the full area of the circle to find what portion of the entire circle is represented by the sector.
\dfrac{ \text{area of sector}}{ \text{area of full circle}} = \dfrac{\dfrac{ 512 \pi}{3 }}{256 \pi }
We can remove the common pi term from the numerator and denominator:
\dfrac{\dfrac{ 512 }{3 }}{256 }
\dfrac{ 512 }{3 } \times \dfrac{ 1}{256 }
\dfrac{ 512 }{768} = \dfrac{ 2}{3 }
So, this means that the sector takes up \dfrac{ 2}{3 } of the entire circle!
We can now use that information to find the measure of the sector angle. We know that a full circle is {360}degree, so the sector angle would be \dfrac{ 2}{3 } of a full circle:
\text{angle of sector} = \dfrac{ 2}{3 } \times 360 degree = 240 degree
The answer choices are given in radians, so we can use the relationship between degrees and radians (provided in the reference sheet) to find our final answer. We are told on the reference sheet:
1\text{ degree}=\dfrac{\pi}{180}\text{ radians}
We are converting 240 degree to radians, so we can multiple the given relationship as follows:
240(1\text{ degree})=240(\dfrac{\pi}{180}\text{ radians})
240 \text{ degrees}= \dfrac{240\pi}{180}\text{ radians})
240 \text{ degrees}= \dfrac{4\pi}{3}\text{ radians})
So, the final answer is Option 2.
Try it Yourself
Test your knowledge of degrees and radians using the Albert practice questions below. Each question includes a detailed explanation so you can continue improving your skills:
- College Trigonometry / Angles, Degrees, and Radians
Return to the Top
Exponential Growth/Decay
A=A_{0}e^{k(t-t_0)}+B_0
What to Know
- A represents the end ending value after the continuous growth or decay
- A_0 represents the starting or initial value before the growth or decay
- e is a constant that serves as the base of the natural logarithm (approximately 2.71828 )
- k represents the continuous rate of growth (or decay)
- t-t_0 represents the amount of time passed where t is the ending time and t_0 is the initial time
- B_0 is any initial starting value before the growth occurred (basically a y-intercept)
- This equation is used on the Algebra 2 Regents exam
When to Use
This formula is used specifically to model continuous exponential growth or decay over a certain period of time.
If the rate of growth ( k ) is greater than 1 , then we have exponential growth because the sequence of values is continuously increasing by a common rate.
If the rate of growth ( k ) is less than 1 , then we have exponential decay because the sequence of values is continuously decreasing by a common rate.
Example question:

Source: August 2019 Algebra 2 Regents, Question 18
The problem above covers concepts of exponential change over time. Since the coffee is cooling at a constant rate of k = 0.05, we would call this “exponential decay”.
To find how long it will take for the coffee to be at a safe temperature of 120 degree , let’s plug in the given values:
F(t) = F_s + (F_0 - F_s)e^{-kt}
120 = 68 + (195 - 68)e^{-(0.05)t}
Subtract 68 from both sides:
52= (127)e^{-(0.05)t}
Divide both sides by 127:
\dfrac{ 52}{127 }= e^{-(0.05)t}
Take the natural log of both sides:
ln(\dfrac{ 52}{127 })=ln( e^{-(0.05)t})
ln(\dfrac{ 52}{127 })={-0.05t}
-0.893 = {-0.05t}
17.86 = t
Rounding to the nearest full minute, our final answer is Option 4.
Try it Yourself
Practice 10 Regents-aligned questions on exponential growth and decay using the Albert topic quiz linked below. If you’re a student, ask your teacher to assign you the quiz so you can test your skills before exam day:
- Algebra 1 / Exponential Growth and Decay (topic quiz)
Return to the Top
Did you find this post helpful?
Check out our other Regents related content:
Regents Study Guides:
- The Best Regents Algebra 1 Study Guide
- The Best Regents Algebra 2 Study Guide
Regents Study Tips:
- Algebra 1 Regents Tips and Things to Remember
- Algebra 2 Regents Tips and Things to Remember
Regents Score Calculators:
- Algebra 1 Regents Score Calculator
- Algebra 2 Regents Score Calculator
- Geometry Regents Score Calculator
Regents FAQ:
- Algebra 1 Regents FAQ
- Algebra 2 Regents FAQ
- Geometry Regents FAQ
